Dear readers, With the launch of e-newsletter CUHK in Focus, CUHKUPDates has retired and this site will no longer be updated. To stay abreast of the University’s latest news, please go to https://focus.cuhk.edu.hk. Thank you.
Geometrically Crispy
Thomas Au motivates his students to explore science from difference perspectives

The ancient Greeks believed that arithmetic and geometry are the soul of music. Even Plato and Aristotle were reluctant to separate mathematics from aesthetics. The works of the Dutch printmaker M.C. Escher exemplify how an artist’s mind makes manifest the beauty in geometry. Kiki Ng, who just graduated this year from the Department of Mathematics and with the help of Prof. Thomas Au of the same department, held the ‘Shining Beauty of Mathematics’ exhibition in front of the Science Centre to pay homage to the inherent beauty in mathematics and art. The geometric floral pattern on the ground of the exhibition zone was inspired by Escher’s Shells and Starfish.
Inherent Beauty in Mathematics and Art
No one would deny the algorithmic precision in mathematics, but few know that its fractal geometric shapes are pleasing to the eye as well. Two of Kiki’s exhibits, a rhombic hexecontahedron and a pentagonal hexecontahedron, drew their inspiration from the concept of regular polyhedron, an important branch of geometry fraught with aesthetic potential. A third exhibit was a fractal display in the form of a Sierpinski triangle. The triangle was made of twisted silver wires, itself encasing a Hilbert Curve (named after the German mathematician who invented it in 1891) of green and yellow aluminum wires. Fractal geometry is closely interwoven into our daily life—leaf veins, blood vessels and brain folds, to name a few, are all expressed in different fractal shapes or fractal-like characteristics.
The artistic Kiki is also a mathematics lover. She has been actively taking part in mathematics competitions since primary school, and aimed at studying mathematics in university. Her favourite quote is from the British mathematician G.H. Hardy: ‘Beauty is the first test: there is no permanent place in the world for ugly mathematics.’ Drawing was Kiki’s way of coping with pre-examination pressures. Last year, she contributed a sophisticated graphic design to the department’s new website—a Klein bottle with refined strokes. With its shape like the mathematical symbol ‘∞’ for infinity, the design welcomes the website visitors to the infinite world of mathematics. To organize an exhibition at the Science Centre had long been Kiki’s dream. When she brought the idea to Professor Au, she was surprised by and grateful for his staunch support: from sponsoring the materials cost to venue arrangement.
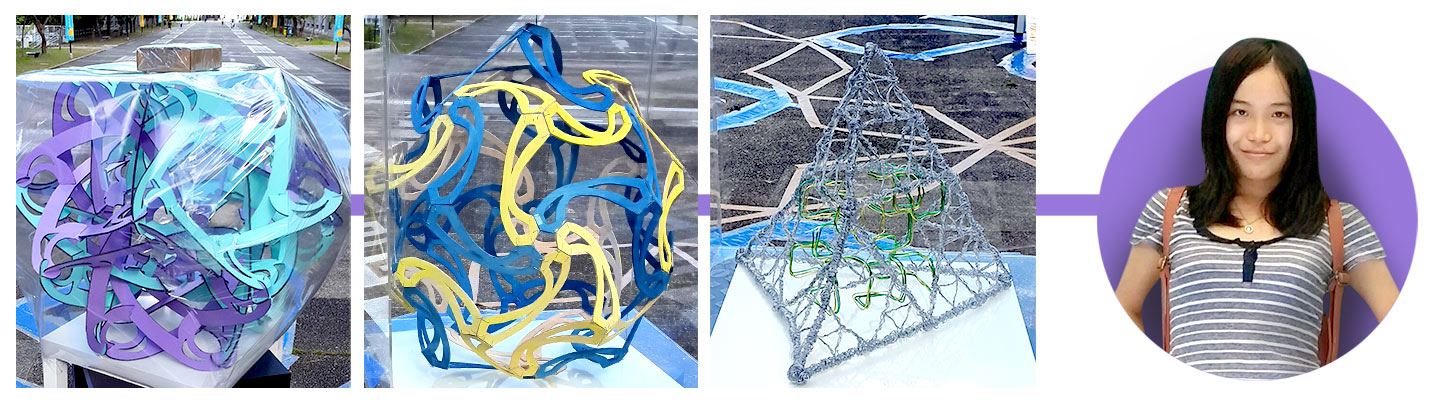
Connecting the Dots to Fathom the Unfathomable
‘Kiki’s exhibition is the kick-off event of the Science Crossover Programme,’ said Professor Au. As the name suggests, the programme supports students of the Faculty of Science to explore the essence of science in non-scientific ways. ‘I encourage the students to be bold in translating their new ideas into proposals. Students who were selected by an interview panel would receive financial support. The funding could be used for organizing exhibitions, producing documentaries, or taking courses relevant to the proposed subjects. On top of grooming students, I wish to unleash their creativity by cross-disciplinary try-outs through which they could deepen their scientific thinking.’ Professor Au reckoned that the scientific exploration itself and the skills acquired from scientific crossover productions would benefit the students for life.
Mathematicians believe that mathematics is the basis of everything. Plato believed that geometric polyhedrons were the origin of the universe. In 1600, a German astronomer came up with an ingenious system of nested Platonic solids to approximate the distances of the known planets from the Sun. ‘Even potato chips embed a mathematically hyperbolic paraboloid: the parabola of a saddle-shape faces upward, and the opposite side faces downward,’ explained Professor Au. ‘The double curvature of a potato chip strikes a delicate balance between different pulls and pushes. The different forces would be spread to the edges of the chip whenever any part undergoes tension or compression, which allows the chip to remain thin yet surprisingly strong.’ Architects also apply the chip principle to evenly distribute the forces buildings are put under, which will all be eventually transmitted onto the ground. Professor Au thought that if the students were keen observers of the things around them, they would begin to appreciate the boundless world of mathematics.

This article was originally published in No. 484, Newsletter in Oct 2016.